Home    Astronomy    Astrophysics

The core temperature of the Sun

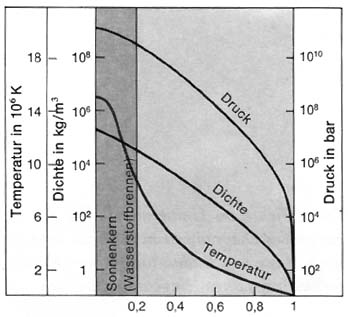
Temperatur im Inneren der Sonne

More

Sun,Sonne,Temperatur,temperature,pressure,Druck,density,Dichte

Pressure, density and temperature inside the Sun
(from Sexl, Raab, Streeruwitz: Materie in Raum und Zeit, Diesterweg/Salle/Sauerländer, 1980)

 

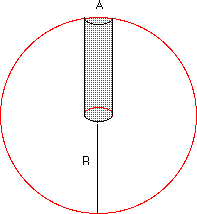
To be in a steady state the thermal gas pressure of the star must be in equilibrium with gravitation. First, we estimate the gravitational pressure in the centre of the star:

star,sun,temperature

The red cylinder (height R, cross-section A) has a mass of

m = ρ·A·R.

Assuming a constant homogeneous density ρ and applying Newton's law of gravity

F = G·m·M / R2

to the surface of the star (radius R) the gravitational force F is

F = G·ρ·A·R·M / R2

M = mass of the Sun
G constant of gravitation
The pressure in the centre of the star is

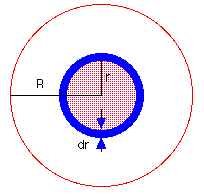
p = F / A = G·ρ·M / R

The ratio p / ρ is given by

p / ρ = G·M / R

On the other hand, the pressure p of the star, considered as an ideal gas of N atoms of mass mA, is

p·V = N·k·T
( k = Boltzmann constant, T = abs. Temperature)

With ρ = N·mA / V we get the ratio

p / ρ = k·T / mA

To be stable the following equation must be valid:

G·M / R = k·T / mA

For the temperature T we get

T = G·mA·M / (k R)

G = 6.7·10-11 N m2 / kg2

mA = 1.7·10-27 kg

M = 2·1030 kg

k = 1.4·10-23 J / K

R = 7·108 m

constant of gravitation

mass of hydrogen atom

mass of the Sun

Boltzmann constant

radius of the Sun

T = 2.3·107 K = 23,000,000 K

A more realistic value is 15,000,000 K (surface temperature: 5800 K)

The pressure in the centre of the Sun

p = ρ·G·M / R

with the mean density ρ = 1.4·103 kg / m3 is

p = 2.7·1014 N / m2 = 2.7·109 bar

The real value should be greater because the density increases towards the center.

 

 

Another calculation for the pressure at radius r

p(r) = F / A

The force of gravity of an infinitesimal layer of thickness dr at radius r (with mass m) caused by the inner sphere (mass M) is

dF = G m M / r2

Inserting

m = ρ·V = ρ·4 pi r2 dr

M = ρ 4 pi/3 r3

we get:

dF = G 4 pi r2 dr ρ2 4 pi/3 r3 / r2

dp(r) = dF / A = dF / (4 pi r2)

= 4 pi/3 G ρ2 r dr

By integration from r to R we find:

The pressure p0 at the centre of the star (r=0) is

p0 = 4 pi/3 G ρ2 R2

With M = ρ·V = ρ 4 pi/3 R3 we get the same result as before:

p = G·ρ·M / R

Web Links

Sun Facts

Our Sun and Stellar Structure (Bakersfield College)

The Sun's Power Source (Bakersfield College)

How the Sun Shines
John N. Bahcall, Institute for Advanced Study, Princeton, NJ

 


Last update 2020, Feb 27