Home Site Map Search

GeoAstro Applets

Astronomy

Chaos Game

Java

Miscel- laneous

large applet version

	Select your time zone offset.
	Enter latitude (northern positive, southern negative), and longitude (eastern positive, western negative) in decimal degrees. Then press "Apply input".

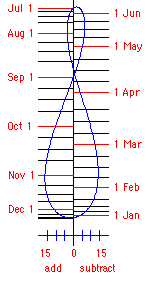
Add or subtract the equation of time to get the standard time.
 

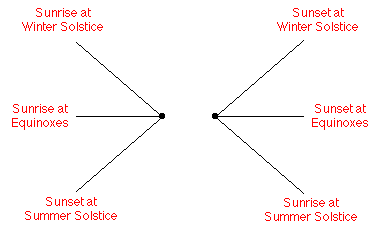
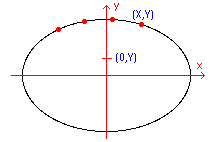
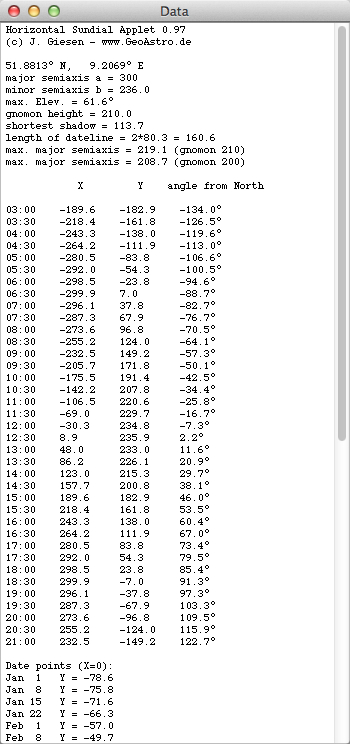
The vertical shadow-casting gnomon is placed on the North-South line depending on the current date. The time is read from the intersection point of the shadow and the ellipse: The height H of the gnomon necessary for the shadow to intersect the ellipse even at summer solstice depends on the observer's latitude. For an ellipse having the major semiaxis a we get latitude H 50° 0.97 a 40° 1.04 a Vice versa, the ratio of maximum length A of the major semiaxis and the gnomon length is shown in the following diagram: latitude A 50° 1.03 G 55° 1.08 G

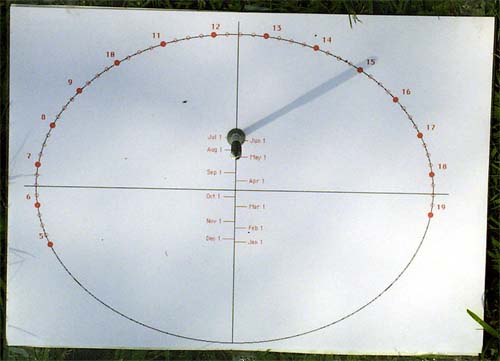
The sundial at my home location (51.62° N, 7.97° E)
2003, June 29 at 12:15 CET
Equation of time is -3m 22s
(sundial is slow, add 3m 22s to the time read from the sundial to get the mean local time)

Use a compass to align the sundial north-south (correction for magnetic declination)
(for my home location the magnetic declination is only 0.1°)

at 15:00 CET

	The figure "8" shape is known as the "analemma". It visualizes the equation of time.
	Select "Show Data" from the Dtails menu to open a window showing the coordinates (X,Y) of the hour points, and the coordinates (0|Y) of the date points:

Sundials for special locations
(North Pole, Arctic Circle, Tropic of Cancer, Equator)

More of my sundials:

Pie Sundial

Analemma Sundial
 

Web Links

Analemmatic sundials: How to build one and why they work (C.J. Budd and C.J. Sangwin) Compute Values of Earth's Magnetic Field (NOAA/NGDC) Analemmatic Sundial Functions (John Carmichael) Of Analemmas, Mean Time, and the Analemmatic Sundial (Frederick W. Sawyer) Make your own Sundial

Last update: 2023, Oct 05