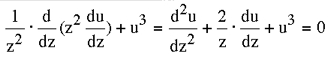Standard Stellar Model

Applet

Wo jetzt nun, wie unsre Weisen sagen,
Seelenlos ein Feuerball sich dreht,
Lenkte damals seinen goldnen Wagen
Helios in stiller Majestät.

aus Friedrich Schillers Gedicht "Die Götter Griechenlands"

The Standard Stellar Model

Emden's differential equation arising in the study of stellar interiors assuming a polytropic model

(constant K, polytropic index n=3) is given by:

Lane Emden differential
equation

u is a (dimensionless) temperature, the (dimensionless) variable z is related to the distance r from the center.

The boundary conditions are:

at the center (z=0, r=0): u=1, du/dz=0,
at the surface (r=R): u(z0)=0.

The solution can only be obtained numerically by my Lane-Emden applet:

solution emden ode polytropic index n=3

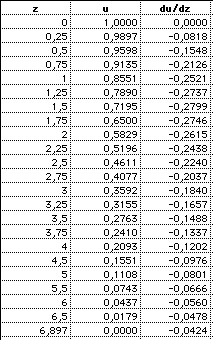
Tools: ODE Toolkit (online), Berkeley Madonna (Mac, Windows trial).

The first zero of u(z) is found to be z0=6.897. The relative distance is r/R=z/z0.

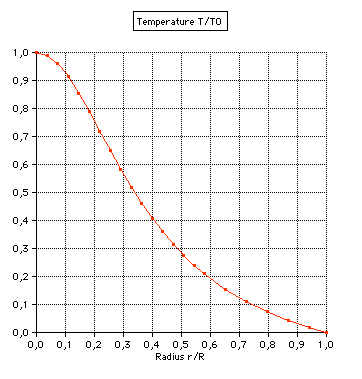
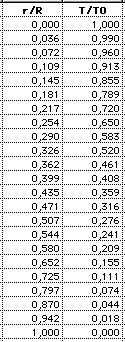
My results for the variables temperature, density, pressure, mass:

(1) Temperature:
T ∼ u

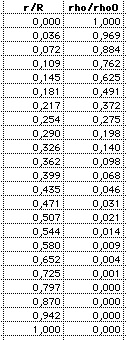
(2) Density:
rho/rho0 ∼ u³

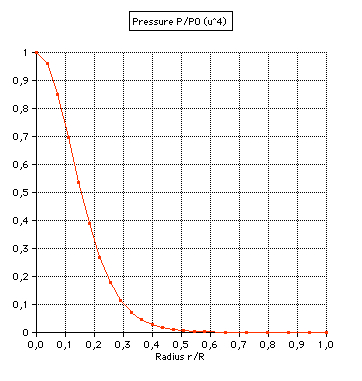
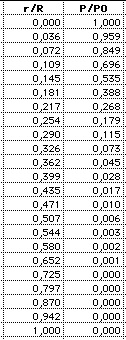
(3) Pressure:
P/P0 ∼ u⁴

(4) Mass:
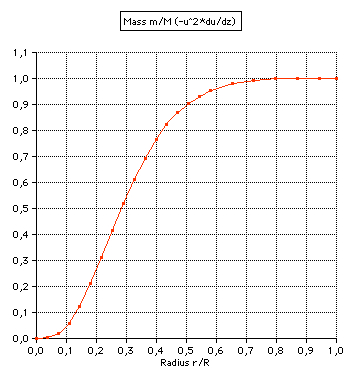
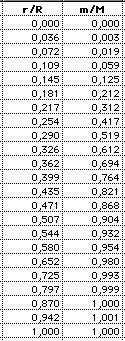
m(r)/M ∼ -u²*du/dz

m(r)/M is the fraction of the total mass within the radius r. About half of the mass is included by the sphere of radius r=0.3*R (2,7% of the total volume).

Relations of the standard model for the core values: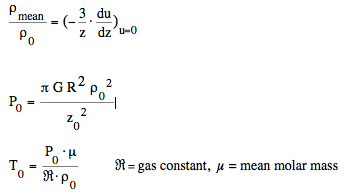

The Sun:

In case of complete ionisation:

Using the mass fractions for the solar composition (Hydrogen x=0.734, Helium y=0.25, heavier elements: 1-x-y = 0.016) the mean molar mass, relative to hydrogen, is:

μ = 0.60114
or, in absolute units:
μ = 0.60114 · 1.0079·10^-3 kg/mol = 6.059·10^-4 kg/mol

The results for the Sun (polytropic index n=3):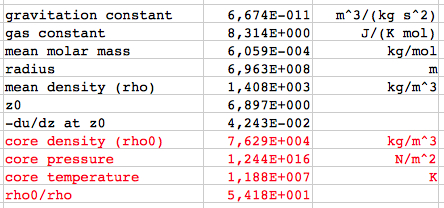

lane-emden result 3

The results for the Sun (polytropic index n=2):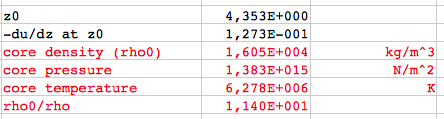

result 2

The results for the Sun (polytropic index n=4):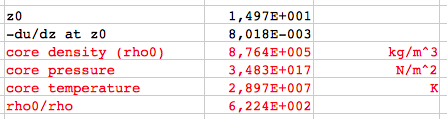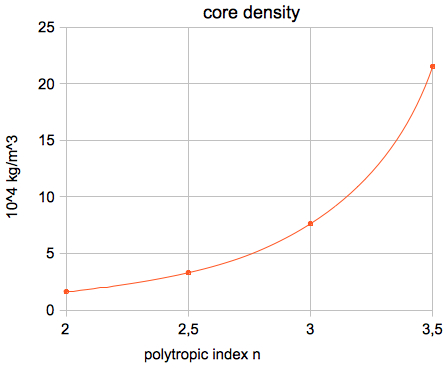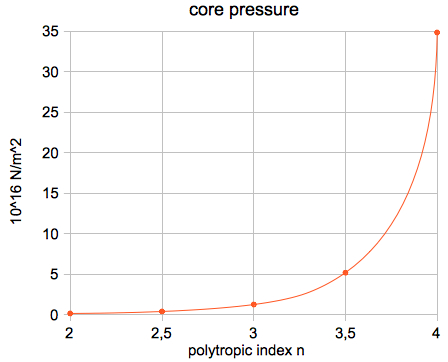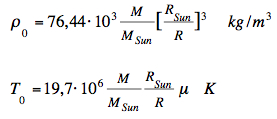

polytropic index 4

In the book of Vogt we find the formulae for the standard model:

which agree very well with the results of my applet.

	Temperature 10⁶ K	Density 10³ kg/m³	Pressure 10¹⁵ N/m²
Lane-Emden model (applet) n=3	12	76	12
Lane-Emden model (applet) n=3.25	14	124	24
Web	15	160
	14	150	23-35
	15.6		34
	15.7
	15.7	162	25

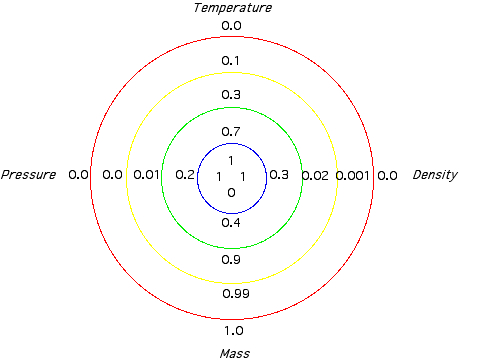
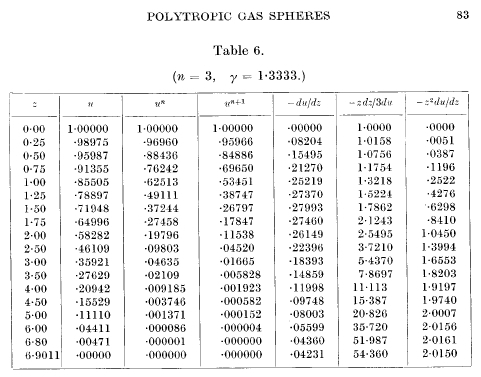Table from the book of Eddington

    "The successive colums give the following physical quantities, expressed in each
    case in terms of a unit which will depend on the star considered:
    1. Distance from the centre.
    2. Gravitational potential. Temperatute (for a perfect gas of constant molecalar weight).
    3. Density.
    4. Pressure.
    5. Acceleration of gravity.
    6. Reciprocal of mean density to the point considered.
    7. Mass interior to the point considered."

Using the symbols g, M, and R for the values at the surface (columns 5, 7, 1):

g = acceleration of gravity = - du/dz
M = mass = -z² du/dz
R = radius = z

we have the relation (a):

(1)

which is Newton's law of gravitation

with gravitational constant G=1 in units of the Lane-Emden equation.

The mean density (column 6) is (b):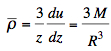

From (a) and (b):